2.1. Classification of flow patterns

Unlike the case of single-phase flow where Reynolds number characterizes the flow completely, thus far, it is not apparent which variable(s) would provide conclusive information. Therefore, the most common method is to develop a flow map for a set of flow conditions and outline the boundaries of different flow patterns (Brauner, 2001a,b). The flow pattern data are generally represented using the fluids volume superficial velocities (Usw = Qw/A and Uso = Qo/A - volumetric flow rate of water and oil respectively/cross sectional area of the pipe). However, prior to construction of flow pattern map, Brauner et al. (1998) proposed that the liquid-liquid system may be classified according to Eötvös number (EOD = ΔρgD2/8σ) as either EOD > > 1 or EOD < 1. EOD > > 1 indicate the flow is gravity dominated and EOD < 1 indicate the flow is surface tension dominated. In general, EOD > > 1 flows correspond to liquids with a finite density difference and sufficiently large tube diameter, where the interface between the fluids is assumed as flat.

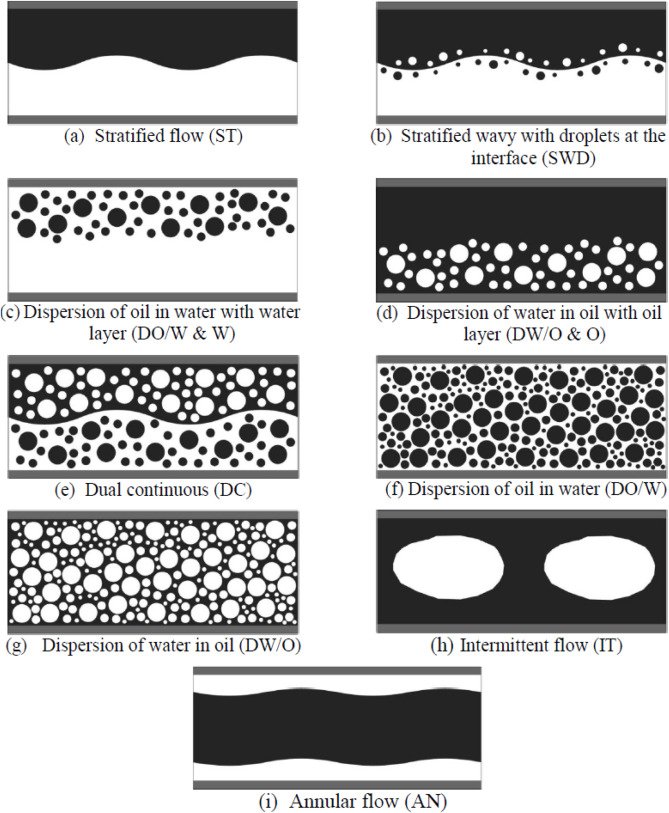
In early research works, uniformity in the nomenclature of the flow patterns was lacking (observed flow patterns are provided in Table 1 below). While reading the flow patterns mentioned in Table 1, discretion is required, since there is possibility of similar flow patterns but described by different nomenclature. Ishii (1975) classified the flow patterns into three categories as Stratified, Mixed and Dispersed. Trallero et al. (1997) classified into three sets of two patterns each. The three sets are: (i) Segregated Flow (stratified flow and stratified flow with mixing interface); (ii) Water-Dominated Dispersed Flow (dispersion of oil in water and water (O/W&W) and Oil in water emulsion (O/W)) and (iii) Oil dominated Dispersed Flow (dispersion of water in oil and oil in water (W/O & O/W) and water in oil emulsion (W/O)), refer Fig. 1. Other researchers like Arirachakaran (1983), Arirachakaran et al. (1989), Nädler and Mewes (1997) used different nomenclature. Brauner (2001a,b) classified the flow patterns into basic four: (i) Stratified (smooth or wavy interface) (ii) Slugs of one liquid into the other; (iii) dispersion of fine droplets of one liquid into the other; (iv) Annular flow (one of the liquids forms the core and the other liquid flows in the annulus). Further, Ibarra et al. (2015a,b) proposed a unified and fundamental flow patterns (Fig. 1) observed in liquid-liquid pipe flow. Another classification of flow pattern was proposed by Torres et al. (2016). They classified four flow patterns with three boundaries. The three boundaries are: (i) Stratified to non-stratified flow; (ii) Transition to semi-dispersed flow and (iii) Transition to fully dispersed flow.

Table 1. Previous studies on liquid-liquid flow in horizontal pipes.

Author(s)	Pipe Diameter (mm)	Pipe material	Viscosity & Density Ratio (oil/water)	Flow patterns observed & Measurement techniques	Inferences
Russell et al. (1959)	20.3	Acetate Butyrate	20.1 & 0.84	STMI, O/W, Visual	1)Flow patterns, pressure gradient and hold-up studied. 2) Flow patterns are independent of oil viscosities
Charles et al. (1961)	26.4	Acetate Butyrate	6.3, 16.8, 65.0 & 1.00	W/O, ANNU, Oil-slugs-in-Water, O/W&W, O/W. Visual	1)Pressure gradient are high for oil continuous flow patterns. 2)Hold-up ratios are greater than one when water is continuous and contact with wall. 3)Addition of water phase in laminar flow lowers the pressure gradient.
Guzhov et al. (1973)	39.4	Steel	21.8 & 0.89	STMI, O/W, W/O, O/W&W, W/O&O/W Visual	1)Flow patterns and pressure drop across the flow is studied.
Kubie and Gardner (1977)	17.0	Acrylic	5.5 & 0.88	W/O, O/W Photography	1)Droplet size distribution and maximum size of drops. 2) Correlations to predict dispersion of drops and drop diffusivity.
Karabelas (1978)	50.4	Acrylic	2.2, 22.2 & 0.80	W/O Photography of encapsulated drops	1)The measured size spectra is represented by either upper limit log-probability function or a Rosin-Rammler form of equation. These equations consider maximum drop diameter and constant parameters.
Ooms et al. (1984)	50.0 203.2	Perspex	1200–3300 & 0.95–0.97	Core Annular Flow (CAF) Visual	1)Only CAF was studied.
Oliemans et al. (1987)	50		3000 & 0.98	Core Annular Flow (CAF) Visual	1)Only CAF was studied.
Arirachakaran et al. (1989)	38.1 25.4	Steel	4.7, 58, 84, 115, 237, 2116 & 0.89, 0.86 0.87	O/W, W/O, W/O&O/W Visual	1)Flow pattern in an oil-water mixture depends on mixture velocity, input water fraction, and oil viscosity. 2) Pressure-gradient prediction models for stratified and for homogeneously dispersed oil-water flows. 3) Correlation for the prediction of the inversion point of oil-water dispersion.
Arney et al. (1993)	16	Transparent PVC & Glass	2700 & 0.99	Core Annular Flow (CAF) & Intermittent	1)Studied liquid holdup for Waxy crude oil. 2) With increase of water volume fraction, nearly perfect core annular flow, wavy core annular flow, and oil plugs in water were observed.
Fujii et al. (1994)	25.0	Transparent Acrylic	55.0 1.00	O/W, W/O, W/O&O/W Plug flow High Speed photography	1)When oil wets the pipe wall, pressure correlation agrees with Lockhart-Martinelli relation.
Kurban et al. (1995)	24.3 24.0	Glass Acrylic	1.6 0.80	ST, STMI, W/O Photography & Conductivity probe	1)Local drop sizes measured. 2) Large fraction of small diameters droplets found.
El-Hamouz and Stewart (1996)	25.0	N/A	10.4 0.80	O/W Laser Diffraction	2)Scanning laser microscope is used to measure drop size distributions 2) Influence of shear by pipes and fittings on the dispersion characteristics. 3) The influence of oil composition is investigated.
Trallero et al. (1997)	50.8	Acrylic Resin	29.7 0.85	ST, STMI, O/W, W/O, O/W&W, W/O&O/W Visual	1)Six flow patterns are classified into two categories. 2)Two fluid model employed to predict fow patterns. 3)Hinze and Levich models are modified to predict droplet sizes.
Nädler and Mewes (1997)	59.0	Perspex	18.0 35.0 0.84	ST, STMI, O/W, W/O, O/W&W, W/O&O/W Visual	1)Various flow patterns observed. 2) Highest pressure gradient observed at phase inversion for water fractions between 10% and 20%. 3) The effect of the temperature is negligible on the flow characteristics.
Vedapuri et al. (1997)	100.0	Plexiglass	2.0 N/A	STMI, W/O&O/W N/A	1)Slip between the fluid phases is relatively less and close to unity.
Beretta et al. (1997)	3.0	Glass	6.17, 4.5, 0.9 & 0.87,0.88	O/W, ANNU, SLUG & Photograph With Stroboscope	1)Flow pattern transition maps are presented. 2)Oil and water flow at the center and periphery of the pipe respectively. 3) Criteria for flow pattern transition is discussed.
Bannwart (1998)	22.5		270 9.89	Core Annular Flow (CAF) Visual	1) CAF was studied
Angeli and Hewitt (1998)	24.3 24.0	Steel & Acrylic resin	1.6 0.80	ST, W/O&O, O/W, W/O, O/W&W, W/O&O/W Conductivity needle Probe	1) Results vary by tube materials. 2) Found influence of wettability of the pipe. 3) Peak in pressure gradient at phase inversion is observed.
Soleimani (1999)	25.4	Stainless steel	1.6 0.80	ST, W/O&O, O/W, W/O, O/W&W, W/O&O/W High Frequency Impedance Probe & Gamma Densitometer	1) Employed Gamma Densitometer to study flow patterns. 2) Water hold-up is higher around the sides of the pipe. 3) In dispersed flow, oil droplets are concentrated at the center.
McKibben et al. (2000)	53	Steel with short glass sections	620–91000 & 0.88–0.99	ST, CAF and Intermittent Visual	1) At low velocities and/or low water fractions, intermittent pattern is observed. 2) Water reduces the pressure drop of heavy oil flow. 3) Water slugs envelop heavy oil.
Angeli and Hewitt (2000a)	24.3 24.0	Steel Acrylic resin	1.6 0.80	ST, W/O&O, O/W, W/O, O/W&W, W/O&O/W Photography & Conductivity needle Probe	1) Compared Steel and Acrylic pipes. Mixed flow pattern appear in the steel pipe at lower mixture velocity. 2) Distribution of the phases differ between the pipes. 3) Average in-situ velocity ratios of the two phases in the acrylic pipe are lower than unity.
Simmons and Azzopardi (2001)	63.0	Acrylic	1.1 0.68	W/O Laser Diffraction (Par-Tec & Malvern)	1) Drop distribution follow Upper Log-Normal distribution. 2) No model describe droplet size at high concentrations.
Elseth (2001)	58.0	Plexiglas	1.6 0.79	SS, SW, SM, Do-DP, Dw-DP, Do-I, Dw-I, Do-H, Dw-H Gamma Densitometer Laser Doppler Anemometer	1) Measurements of local phase fractions, pressure drops, slip ratios and velocity and turbulence distributions. 2) It was found that two-fluid model prediction is best for intermediate water cuts. (In high water fraction, two-fluid model is not satisfactory). 3) Interface dampen the turbulence in the flow.
Liu et al. (2003)	40.0	Plexiglas and Stainless Steel	428.0 0.89	ST, STMI, O/W, W/O, O/W&W, W/O&O/W, SLUG Visual	1) Flow pattern and its transition are studied. 2) Semi-theoretical correlations are proposed for transition of flow patterns. 3) Pressure drop is measured.
Lovick and Angeli (2004a,b)	38.0	Stainless steel	6.0 0.82	ST, O/W, W/O, (O/W&W, W/O&O/W Studied) Impedance probe	1) Pressure drop is less than that of single oil phase flow. 2) Two-fluid model do not predict the pressure drop for dual continuous flow.
Wegmann and Rudolf von Rohr (2006)	5.6, 7.0	Glass	4.3, 5.2 & 0.82	ST, ANNU, O/W, W/O, Intermittent High Speed Camera	1) In smaller pipe interfacial tension forces dominate gravitational forces. The low viscosity fluid phase wet the whole periphery of the pipe. 2) Occurrence of intermittent flows increases with smaller diameter pipe.
Mandal et al. (2007)	25.0, 12.0	Polymethyl - Methacrylate (PMMA)	1.2 0.79	SS, SW, SLUG, PLUG, Dual Continuous, Rivulet, O/W, Visual, Digital Photography	1) Influence of pipe diameter on flow pattern. Rivulet flow and Churn flow are noticed in the smaller diameter pipe. 2) Effect of surface tension increase in the narrow pipe. 3) Design of mixer influence the distribution of the two fluid phases.
Al-Wahaibi and Angeli (2007)	8.0	Stainless steel	5.5 0.82	ST,SW Conductivity probe High speed camera	1) Model to calculate critical wave amplitude was developed from Kelvin–Helmholtz instability. 2) Required entrainment wavelength over the pipe diameter reduces as the oil viscosity increase.
Vielma et al. (2007)	50.8	Transparent pipe	15.0 0.85	ST, STMI, O/W, W/O, O/W&W, W/O&O/W Conductivity probes and High speed camera	1) Experimental data on flow patterns, pressure drop, hold-up, phase distribution and droplet size distribution. 2) Log normal distribution represents droplet size data for dispersions and bimodal distribution for dual continuous flow.
Sotgia et al. (2008)	21–40	Plexiglass and Pyrex	919 & 0.89	ST, CAF, Intermittent, O/W	1) Oil-in-water dispersion and Core Annular Flow were the important flow patterns. 2) Slugs/plugs of oil-water were observed.
Grassi et al. (2008)	21	Polyarbonate	800 & 0.89	ST, CAF, Intermittent, O/W	1) Core annular flow and oil in water dispersion were the important flow patterns. 2) Slugs/plugs of oil-water were observed.
Vuong et al. (2009)	50.8	Stainless Steel	220, 1070 0.88	O/W High Speed Camera Photography	1) A new flow pattern with dispersion of O/W with thin film. No significance of viscosity of oil flow patterns. 2) Oil viscosity, flow rates effect pressure gradient.
De et al. (2010)	12.0	Persplex	1.2 0.79	Rivulet Visual & Photography	1) Oil phase move as a spiral stream with high degree of interfacial waviness. 2) Either phase can wet the pipe wall with greater tendency for kerosene to be at upper portion of the pipe. 3) Holdup and pressure drop are compared.
Kumara et al. (2010)	56.0	Stainless Steel	1.64 0.79	ST, STMI, O/W, W/O, O/W&W, W/O&O/W Visual, Gamma densitometer, PIV	1) Instantaneous velocity measurements of the flow. 2) Instantaneous local velocities, mean velocities, root mean squared velocities and Reynolds stresses are calculated.
Xu et al. (2010)	20.0	Acrylic resin	3.0, 0.85	ST, STMI, O/W, W/O, O/W&W, W/O&O/W High Speed Camera	1) Flow pattern transition and hold-up fraction is studied and verified with existing two-fluid model.
Al-Wahaibi and Angeli (2011)	8.0	Stainless steel	5.5 0.82	ST,SW Conductivity probe High speed camera	1) At the beginning of entrainment, the mean amplitude and length are found to be a function of the velocity between the fluid layers and of the turbulence in each layer.
Al-Awadi (2011)	26	Perspex	3840–16000 & 0.91–0.94	Core Annular Flow (CAF)	1) Transitional flow regime between oil-continuous and water-continuous flow. 2) Flow structures in water continuous further classified into core annular flow, oil plugs in water and dispersed oil lumps in water.
Pouplin et al. (2011)	50.0	Poly Methyl Methacry-late	8.0 0.62	W/O, O/W PIV	1) Flow regimes are identified using particle image velocimetry. 2) Friction factor follow Hagen-Poiseuille for laminar flow and Blasius' law for turbulent regimes. 3) Transition to turbulence gets delayed with the increase of dispersed fluid phase fraction.
Wang et al. (2011)	25.4	Stainless steel	628 0.94	W/O Dispersed, W/O & partial segregated, W/O&W Startified, W&W/O semi Annular, W&W/O Annular Visual	1)For low water fractions, flow is dispersed. 2) Pressure drop decrease when water separates from the oil continuous phase. Pressure drop is higher in crude oil than in model oil.
Al-Wahaibi et al. (2012)	19.0 25.4	Acrylic	6.4, 12.0	ST, STMI, DUAL CONTINUOUS Visual High Speed camera	1) Pipe diameter effects the transition from stratified to non-stratified flows. 2) Experimental amplitudes at the transition agree with the proposed critical amplitude model. 3) None of the available models predict the transition.
Rodriguez and Baldani (2012)	26.0	Borosilicate glass pipe	280 8.28	ST Optical Tensiometer, Rheometer and High speed camera	1) New closure relation for the interfacial friction factor is suggested. 2) It was found that for the slower lighter phase (oil) the effective wall friction factor is significantly lower than the single-phase friction factor
Morgan et al. (2012) and Morgan et al. (2013)	25.4	Stainless steel	2.3 0.79	ST, STMI, O/W, W/O, O/W&W, W/O&O/W Laser-induced fluorescence (LIF)	1) At low oil fractions, interface separating the two phases is not affected. 2) Mean droplet size increase and is largest at intermediate and smallest at high superficial velocities.
Plasencia et al. (2013)	16.0, 32.0, 60.0, 90.0	Acrylic	1.8, 10.0 With surfactant	W/O, Conductance probe, Gamma Densitometer, Focused Beam Reflectance Technique	1) Pressure drop and droplet sizes are measured. 2) Effective viscosities calculated using pressure drop. iii. Above 40% water fraction, effective viscosity increases.
Alagbe (2013)	26	Perspex	3700–7100 & 0.91–0.94	Core Annular Flow (CAF) Visual	1) Transitional flow regime between oil-continuous and water-continuous flow. 2) Flow structures in water continuous further classified into core annular flow, oil plugs in water and dispersed oil lumps in water.
Edomwonyi-Otu and Angeli (2014)	14.0	Acrylic	5.50 0.82	ST, SW, RF, O/W&W, O/W, W/O Photography & Conductivity needle Probe	1) Correlation to predict the height of two phases is developed. 2) Curvature Interface and equivalent roughness calculated using the modified two-fluid model enhanced predictability of pressure gradient and height of interface.
Ibarra et al. (2015a,b)	32.0	Acrylic	6.1 0.82	ST, STMI, O/W, W/O, O/W&W, W/O&O/W High Speed photography	1) Inlet effects on flow patterns, pressure drop and hold-up phase fractions are analyzed. 2) Plate at inlet was kept to generate transition to stratified flow. When the height of plate match with the hold-up water fraction height at low water fraction.
Shi (2015)	26	Perspex	3300–16000 & 0.91–0.94	Core Annular Flow (CAF) Visual	1) Transitional flow regime between oil-continuous and water-continuous flow. 2) Flow structures in water continuous further classified into core annular flow, oil plugs in water and dispersed oil lumps in water.
Smith et al. (2015)	69.0	Steel	173.0, 186.0 0.87	W/O, Continuous water layer, Continuous oil layer, Emulsion layer with continuous water	1) New point model for water–oil flow is developed. 2) Oil–wall shear stress is adequately predicted using new model.
Ibarra et al. (2016) and Ibarra et al. (2018)	32.00	Acrylic pipe	5.5 & 0.83	Stratified Flow Stratified Wavy Flow	1) Planar Laser-Induced Fluorescence (PLIF) and Particle Image and Tracking Velocimetry (PIV/PTV). 2) rms velocity is high in shear regions of pipe wall and oil-water interface.
Schümann et al. (2016)	100.0	–	35.0, 60.0, 120.0 0.85,0.86,0.87	O/W & W/O Focused Beam Reflectance Technique	1) Droplet sizes is function of the dispersed phase fraction and increase to a maximum at phase inversion. 2) Existing models over-predict the present droplet size data.
Voulgaropoulos et al. (2016)	37.0	Acrylic Pipe	5.5	ST, STMI, O/W, W/O, O/W&W, W/O&O/W High Speed photography Conductivity Probe	1) Flow remains fully dispersed downstream the inlet at high mixture velocities. 2) At low velocities, an accumulation of dispersed drops at the upper part of the pipe. 3) Drop size depend on the spatial configuration of the flow.

 

Abbreviations used in Table 1. ST – Stratified Flow, SS – Stratified Smooth, SM, STMI – Stratified Flow with Mixing Interface, SW – Stratified Wavy, RF – Rivulet Flow, ANNU – Annular Flow, Dw-DP, O/W&W – Dispersion Oil in Water and Water, Do-DP, W/O&O – Dispersion Water in Oil and Oil, W/O&O/W – Dispersion Water in Oil and Oil in Water, Do-I – Dispersion of water in oil – inhomogeneous, Dw-I – Dispersion of oil in water – Inhomogenuous, Dw-H, O/W – Dispersion Oil in Water and Do-H, W/O – Dispersion Water in Oil.

 

1. Download : Download high-res image (1MB)
2. Download : Download full-size image

Fig. 1. Schematic of flow patterns in horizontal flow (adapted from Ibarra et al., 2015a,b).

Recently, to investigate the effect of mixing at the entry, experiments were carried out with mineral oils of viscosity (120 mPas; 60 mPas; 35 mPas) and water by Schümann et al., 2016a, Schümann et al., 2016b. The water fractions employed were 0%–100% with a mixture velocity of 1.1 m/s. Oil and water were mixed at the entry using a static mixer. They observed that the mixing at the entry enhances the transition to dispersed flow and transition occurs at lower velocities.

Other methods to classify flow patterns were: (i) using mass-flux (m/A) proposed by Hapanowicz (2010) and (ii) recently proposed non-dimensional parameters (Rem/Eo) by Ibarra et al. (2015a,b). They included the dimensionless parameters representing the governing forces of the flow, i.e., inertial, viscous, gravitational and surface tension (Fig. 2). In Fig. 2, Rem refers to Reynolds number based on mixture velocity and Eo is EÖtvos number. Water cut is defined as the ratio of volume of water phase to the total volume of both fluid phases combined.

1. Download : Download high-res image (220KB)
2. Download : Download full-size image

Fig. 2. Non dimensional Flow pattern classification by Ibarra et al. (2015a,b).

Zhai et al. (2015) employed mini-conductance probes to investigate the oil-water horizontal flow structure for various flow conditions. The probes were able to identify the evolution of the flow structure. Also, they compared the transitional boundaries (both experimental and theoretical) and found that the model proposed by Trallero et al. (1997) was poor in calculating the boundary from ST to ST&MI pattern.

To investigate the dynamics of oil-water flow, Ibarra et al. (2016) and Ibarra et al. (2018) conducted experiments in a 32 mm horizontal pipe. Simultaneous two-line laser-based, Planar Laser-Induced Fluorescence and Particle Image Velocimetry, were used to study the interfacial interaction, and velocity profiles(axial and radial) and velocity fluctuations. Experiments indicated peaks in high shear regions like near pipe wall, at liquid–liquid interface and inside the oil layer. The results also demonstrated that the velocity fluctuations are higher in the oil phase than the water phase.

The flow patterns observed for high viscosity oil-water flow differ markedly with the flow patterns for low viscosity oil-water flow. In general, in high viscosity oil-water flows, in addition to the Core Annular Flow (CAF), intermittent pattern containing oil slug/plugs, large oil drops in water and dispersed oil in water are the prevalent flow patterns (Fig. 1). For example, Yang (2014), for viscous oil, did not observe the flow pattern of dispersed water-in-oil with a continuous oil layer. When the water is the continuous phase, there is negligible effect of oil viscosity on flow patterns. Research works, exclusively, for high viscosity fluids are Ooms et al. (1984), Oliemans et al. (1987), Bannwart (1998), McKibben et al., 2000a, McKibben et al., 2000b, Liu et al. (2003), Sotgia et al. (2008), Grassi et al. (2008), Vuong et al. (2009), Zhang et al. (2010), Sridhar et al. (2011), Al-Awadi (2011), Wang et al. (2011), Rodriguez and Baldani (2012)and Shi (2015).

Recently, Shi and Yeung (2017) indicated the absence of viscosity in the classification of flow using Eötvös number. Hence, they proposed a new method of classification by introducing viscosity, i.e. the ratio of the gravitational forceto viscous force, $\raisebox{1ex}{$$}\!\left/ \!\raisebox{-1ex}{$$}\right.\frac{{}^{\mathrm{}}}{}$. They demonstrated that liquid-liquid flows as: (1) Stratified and Dispersed patterns are gravity dominant and characterized by high G/V; (2) Core Annular Flow and Intermittent patterns are viscous dominant with low G/V and (3) Stratified, Dispersed, Core Annular Flow and Intermittent are comparable gravity and viscous force with medium G/V. Analyzing experiments with high viscous oil, they observed that the flow is gravity dominant at G/V ≈ 1. And the transition from gravity dominant to viscous dominant occurs at G/V ≈ 40. Effectively, using Eötvös number and G/V relation, it can be determined whether flow is either gravity dominated or surface tension dominated or viscosity dominated. A schematic of flow patterns of high viscous oil-water horizontal flow are provided by Shi and Yeung (2017).

When there is low density differential between the two-fluids, in liquid-liquid flows, the effect of gravity is reduced. And wetting and surface tension properties exhibit significant effect on the flow pattern (Cai et al., 2012). Consequently, in stratified flow, the interface between the fluid phases is not planar but either concave or convex (Valle and Kvandel, 1995; Angeli et al., 2002; Ng, 2002; Gat, 2002; Ong et al., 1994). When core fluid phase detach from the wall, Core Annular Flow (CAF) pattern is formed. Thus, the occurrence of annular flow (CAF) is therefore observed in liquid-liquid flows with less density differential and small diameter pipes (characterized by Eötvös number). The CAF pattern results in huge reduction in the pumping power during the transport of heavy viscous oil. Though CAF is preferred flow pattern for transportation, this type of flow pattern is rarely observed in practice (Bensakhria et al., 2004). However, in practice, CAF can be established for a particular range of water fraction and using suitable inlets (nozzle). Further, Morgan et al. (2016) investigated development of immiscible liquid–liquid flows by introducing the denser phase at the top of the pipe and above the less dense phase. They evaluated the interfacial instabilities and its effect on the flow patterns and characteristics.

2.2. Flow pattern maps using momentum/mass-flux

As mentioned earlier, superficial (volume) velocities of the fluid phases are generally used to map the flow patterns. Only recently, Hapanowicz (2010)analyzed superficial (mass) velocities (ρQ/A) of oil and water using the data from literature for systems with different fluid phase properties. This analysis is similar to Hewitt and Roberts (1969), for gas-liquid flows, wherein they developed plots with gas momentum flux against liquid momentum flux. On the map, Hapanowicz (2010) drawn boundary “arbitrarily” to differentiate the two types of dispersions (water dominant and oil dominant) and developed criterion to identify the type of dispersion system.