. Introduction
One of the key challenges in carbonate fractured reservoir engineering is the concept of ‘block to block interaction’. Both laboratory experiments and numerical analysis have been carried out by different researchers to understand this phenomenon and its mechanism.
1.1. Gravity drainage
Dumore and Schols (1974) reported that due to gravity drainage in high permeability sandstone core, the oil residual saturation could be as low as 5%. Clemens and Wit (2001) showed that the drainage rate is further reduced if there are no fractures in the horizontal plane, and the oil has to flow sideways towards the fracture system resulting in lower drainage rates. Karimaie and Torsaeter (2008) performed experiments to investigate gas injection in fractured carbonate rock. They mainly investigated the effect of gas injection on gravity drainage process. This study showed that the recovery of oil increased significantly by re-pressurization in gravity drainage process. They also demonstrated that low-IFT (Inter Facial Tension) gravity drainage is capable of recovering a significant portion of oil in fractured reservoirs even after water injection. Boerrigter et al. (2007) presented a dual permeability simulation formulation capable of handling GOGD (Gas-Oil Gravity Drainage) and expansion imbibition processes, impact of fracture-spacing, block-height, GOGD of sub grid-block effects, re-imbibition effects and interaction of GOGD with EOR processes.
1.2. Re-infiltration
Saidi et al., 1979a, Saidi et al., 1979b studied the process of block to block flow within a column of matrix blocks that were not in contact with each other. They concluded that the recovery rate under this system was much slower than that in a group of independent single blocks. Saidi (1987) speculated that the re-infiltrated oil prefers to pass through the saturated block without displacing the original oil. Saidi et al., 1979a, Saidi et al., 1979b and Firoozabadi et al. (1991)described the behavior of stacked layers separated by shale or other flow barriers under simultaneous drainage. They found that in such system, the overall production rates are lower due to re-imbibition of the oil into the bottom layers. Vidal (1992) performed gravity drainage experiments to study the drainage and re-infiltration process using two porous media with different porosity and permeability. Firoozabadi and Ishimoto (1994), using one-dimensional modeling of re-infiltration process in fractured porous media, showed that all drained oil from upper matrix block is discharged into lower matrix block and does not flow through the fracture network. They found that the effect of fracture geometry on re-infiltration process is negligible. Firoozabadi et al. (1994) presented two dimensional modeling of re-infiltration process in a tilted block. The results of their modeling showed that there is considerable variation in re-infiltration rate across a vertical face of a matrix block. Moreover, the results of their work showed that re-infiltration could be effective in a small area at the top portion of a matrix block. Saidi (1987) and Sajjadian et al., 1999a, Sajjadian et al., 1999b experimentally investigated the effect of fracture angle variation on the re-infiltration process. The results of their works showed that the re-infiltration process depends on the physical properties of the matrix blocks, the rate of oil supplied the drainage of the blocks. Sajjadian et al., 1999a, Sajjadian et al., 1999b performed laboratory studies of gravity drainage in fractured rock investigating the effect of capillary continuity and re-infiltration. The results of their experimental work discussed the effective parameters on re-infiltration process. Dejam et al. (2009) worked on the impact of fracture angle and fracture aperture variation on re-infiltration process through discrete traveling liquid elements and continuum film flow along inclined fractures. They derived two algorithms for oil velocity based on discrete traveling liquid elements and liquid film flow along inclined fractures, using analogy from hydrology and fluid mechanics. Their results showed the effect of the geometry of fractures between matrix blocks and the fracture aperture or fracture dip angle on re-infiltration process.
1.3. Mixing in fractures
Hagoort (1980) presented analytical solutions for an immiscible gas/oil drainage flow in a homogeneous stack in the absence of capillary pressure. Verlaan and Boerrigter (2006) discussed the behavior of immiscible GOGD (Gas Oil Gravity Drainage) as compared to fully miscible gas through modeling the behavior of a matrix block. Tan and Firoozabadi discussed that the absence of re-imbibition in miscible GOGD has a major impact on the oil production from heterogeneous stacks.
Zobeidi et al., 2015a, Zobeidi et al., 2015b describe the results of several experiments that were carried out to investigate the block to block interaction in fractured limestone reservoirs for both gas/oil and solvent oil systems by use of carbonate cores. The focus of this research was to understand gravity drainage, reinfiltration, and mixing in fractured systems with a set of laboratory experiments. Also Zobeidi et al., 2015a, Zobeidi et al., 2015b describe the simulation of one-phase miscible gravity drainage performance of a stack of matrix blocks.
2. General field data
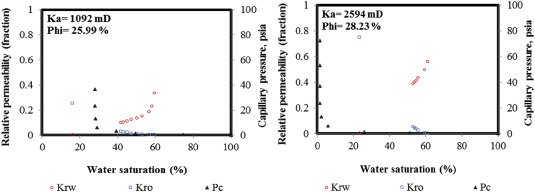
The oil field of interest is located in South west of Iran in Zagros fold belt. Two major types of lithofacies have been observed in the reservoirs: carbonate (mainly lime stones and dolomites) and anhydrite. Special Core analysis results indicated that the core is water wet to mix wet. The fracture density of this reservoir is in the range of 0.42–16.76 per meter with an average 1.3, the fracture aperture is in the range 0.1–0.62 mm and its average fracture porosity is 0.0012%.Typical SCAL results are shown Fig. 1.
Fig. 1. Typical oil-water relative permeability, and capillary pressure curves for rocks of similar permeability.The reservoir fluid properties are summarized in Table 1.
Table 1. Reservoir fluid properties.
| Reservoir and PVT data | Composition of crude oil (%) | ||
|---|---|---|---|
| Initial pressure | 3555 PSIA | C1 | 40.3 |
| Temperature | 135.4 °F | C2 | 6.3 |
| Saturation Pressure | 2723 PSIA | C3 | 5.7 |
| BO | 1.255 vol/vol | C4 | 5.0 |
| Rs | 573.5 scf/bbl | C5 | 4.6 |
| API | 36.4∘API | C6 | 3.5 |
| Viscosity | 0.727 cp | C7+ | 33.0 |
3. Experimental procedure
In order to address key production mechanisms in this reservoir, several experiments were carried out to study infiltration and gravity drainage (see Table 2). The carbonate blocks had an average porosity of 21.5% and an effective permeability of 2.7 darcies. This high permeability can be attributed to presence of natural fractures within the matrix. In the first series of tests, oil was added to the top of a tilted block or stack of blocks to measure the infiltration rate. In the second series of tests, the carbonate blocks were submerged in gas to investigate the gravity drainage rate. In the third series of tests, oil was added to the blocks post gravity drainage. Finally, in the fourth series of tests, gas or solvent was injected in a stacked column to measure the oil recovery due to gas displacement. Notice that although initial water saturation was not established in some of these tests, we have used the term “imbibition” to describe the process of water infiltration into the core. These tests are discussed below:
Table 2. Experimental conditions.
| Description | Equipment | Injection Conditions | Type | Matrix pore volume, cc |
|---|---|---|---|---|
| oil imbibition into tilted matrix blocks | Limestone cores | kerosene addition air surrounded | one cylindrical core | 107 |
| kerosene addition air surrounded | stack of 4 cylindrical cores | 428 | ||
| gravity drainage in blocks | Limestone cores | kerosene addition gas surrounded | one cylindrical core | 656 |
| kerosene addition gas surrounded | two cylindrical cores | 1312 | ||
| imbibition/gravity drainage in blocks | rectangular Limestone blocks | kerosene addition solvent surrounded | two blocks | 64.5 |
| miscible and immiscible injection with gravity drainage | Limestone cores | equilibrium oil/gas drainage | one cylindrical core | 107 |
| enriched gas injection | ||||
| equilibrium oil/gas drainage | four cylindrical cores | 428 | ||
| enriched gas injection |
4. Oil imbibition (infiltration) into a tilted matrix block
The first series of experiments were designed to investigate the infiltration rates of oil into a limestone block under continuous oil addition. The blocks were cylinders of 9.3 cm diameter and of different heights (average 7.4 cm) as shown in Fig. 2.
Fig. 2. Schematic of experimental setup for miscible gravity drainage in a stack of four blocks.The blocks were originally fully saturated with kerosene while submerged in the oil. At the moment when the blocks were surrounded by air, oil was supplied at the top of the blocks using a syringe. The addition of this oil was done to simulate the liquid bridges that are formed within fractures between blocks (to be discussed later). A ‘wet’ region on top of the block then enabled the oil to be imbibed into the block (See Fig. 2). The relation between the size of the wet region and the rate of oil supply was determined for different block heights; various tilt angles of the block and for different locations of the wet region.
In order to understand the effect of tilt angle on re-infiltration process, a block of 13 cm in height was used. The block height was less than the capillary rise between oil and air and consequently the block always remained liquid filled. Several measurements were carried out with the experimental set up shown in Fig. 2. With the top surface of the block in horizontal position and oil being supplied at its center, the ‘wet’ region was a circle around the needle. The diameter of the wet region increased with increasing rate of oil supply. When the rate of oil supply was equal to the maximum possible drainage rate of the block (without capillary retention forces), the total top surface was just covered by thin liquid film. This maximum drainage rate is given by:(1)qmax=(Δρo/g.g.k/μo) × S
When the block was tilted, the wet region assumed a more or less elliptical shape and the oil was now supplied near the highest point of the top surface. Table 3 gives the results of the experiments. The size of the wet region, Swet, is expressed as a fraction of the total cross-sectional area of the block denoted by S, while the rate of oil supply q is expressed as a function of qmax. For the cases where the block was tilted, also the eccentricity (ratio between the long axis and the short axis) of the elliptical wet region is given.
Table 3. Relation between the size of the wet region and the rate of oil supply for different tilt angle α
| q/qmax | Eccentricity | ||||
|---|---|---|---|---|---|
| α = 0° | α = 1.3° | α = 5.1° | α = 11.5° | ||
| oil addition at the center | oil addition near the side boundary | ||||
| 0.16 | 1% | – | – | – | – |
| 0.23 | 2% | 2.5% | – | 2% (1.5) | 1% (2.7) |
| 0.29 | 4% | 5.5% | 5% (1.2) | 3.5% (2) | 2.5% (3.4) |
| 0.47 | 19% | 24% | 23% (1.4) | 15.5% (3.8) | overflow |
The results showed that a relatively small wet region allowed a considerable infiltration rate. When the oil was supplied near the block boundary with the top surface in horizontal position, the required wet region was somewhat larger than when the oil was supplied in the center of the block. Comparing the tilted cases with the horizontal one, it appears that the infiltration for an elliptical shape of the wet region was more effective than for a circular wet region.
With a tilt angle of 11.5° and a rate of oil supply of 0.47 qmax, the length of the wet region increased to such an extent that part of the oil reached the side boundary opposite the syringe location and started to flow downwards along the side boundary. However, the oil did not reach the bottom of the block, but was imbibed into the block via the side boundary. Only when the rate of oil supply approached qmax did the liquid film along the side boundary reach the bottom of the block.
4.1. Effect of block height
To determine the effect of block height on the infiltration process, the oil addition was carried out to both a 48-cm long block and a 13-cm carbonate rockcore. Since 48 cm is more than the gas/oil capillary rise, the oil supply rate was kept above 0.34 qmax. In order to prevent the block from quick drainage, it remained completely liquid filled during the experiment. Table 4 shows a comparison of the infiltration rates for 13-cm and 48-cm blocks with different wet region sizes:
Table 4. Relation between the size of the wet region and the rate of oil supply for two different block heights.
| Swet/STotal | qinfiltration/qmax | |
|---|---|---|
| H = 13 cm | H = 48 cm | |
| 1% | 0.16 | 0.50 |
| 2% | 0.23 | 0.61 |
| 4% | 0.29 | 0.76 |
| 19% | 0.47 | 0.86 |
For equal sizes of the wet region, the longer block had higher infiltration rates than the shorter one. When the oil was added at a rate equal to 0.5 qmax, the 13 cm block showed a wet region of 19%, while the wet region for the 48 cm block was only 1% of the total cross sectional area. Comparing the results for two different block heights, we may conclude that the infiltration into a block becomes more effective with increase in block height.
4.2. Oil addition to the top of the side boundary of a block
During the experiments with the tilted 13 cm block, it was found that the infiltration of oil into the block could happen through the side boundary, even if the block was filled fully with liquid. In Table 5, the results of the case where oil was added to the center of the top surface are compared with the case where oil was added to the side boundary. The ratios of length and width (eccentricity) of the wet region for these two cases are also shown in this table.
Table 5. Relation between the size of the wet region (expressed as a fraction of the horizontal cross sectional area) and the rate of oil addition at the top and at the side of the block.
| qinfiltration/qmax | Swet/Scross section (Eccentricity) | |
|---|---|---|
| Oil addition at the top | Oil addition at the side | |
| 0.50 | 1% | 1% (2.0) |
| 0.61 | 2% | 2.5% (2.5) |
| 0.76 | 4% | 9% (4) |
| 0.86 | 19% | 16.5% (6) |
| 1.00 | 100% | 60% (14) |
The results suggest that only a small wet region was sufficient to allow high infiltration rates. For low values of eccentricity (ratio between length and width of wet region) the infiltration in the center of the top surface was more effective than at the side boundary. At greater eccentricity of the wet region, the infiltration through the side boundary became more effective. These two findings are in line with the results obtained with the 13-cm block (see Table 3) for different tilt angles. The infiltration rate through the side boundary seems to be high enough to prevent the oil from flowing from top to bottom along the vertical side boundary. The supplied oil was imbibed before reaching the bottom of the block.
It is assumed that the capillary forces are responsible for these processes in order to make the infiltration an imbibition process. However, the infiltration is also active in a fully liquid saturated block. In the wet region, the capillary pressure between the oil and gas is zero. The same holds for the bottom surface of the block. This means that there is a difference in oil potential between the wet region and the bottom of the block. This potential difference is equal to Δρo/g.g.H, where H is the block height. This gravity potential difference causes the oil to flow.
5. Gravity drainage in blocks
In the second set of experiments, the gravity drainage performance for a single block was measured when it was instantaneously surrounded by gas at time zero. The block was 48 cm in height, 9.3 cm in diameter and 63.585 cm2 cross sectional area. The porosity was 21.5% and the pore volume was 656 cc. Using Eq. (1), the maximum drainage rate (without capillary retention) for the kerosene-oil was estimated to be 0.135 cc/sec.
Fig. 3 gives the cumulative block recovery as a function of time. The time is expressed in a dimensionless form t/T = tD, where T represents the time to recover the total block pore volume at a rate:(2)qmax = Δρg.ok /μo.πrb2
Fig. 3. Comparison the values of the reality and the conceptual of the gravity drainage.T is therefore defined as(3)
The ultimate recovery was 27% of the oil in place. Assuming that the residual oil saturation in the gas zone is 20% (an average value for this kind of carbonate rock), the effective capillary rise was estimated to be 66% of the block height. For this capillary rise one would expect an initial drainage rate of 0.34 qmax (see Fig. 3). In the experiment the initial gravity drainage rate was 0.05 cm3/s and this was equal to 0.37 qmax. The initial gravity drainage rate was therefore in line with the capillary retention effect.
By differentiating the recovery with respect to time, we obtained the gravity drainage rate as a function of time. This relation was used for the rate of oil addition to the top of a block that was initially liquid filled. The block was tilted at an angle of 5° to allow the oil to flow along the top surface, in case the infiltration rate was not high enough. The infiltration was so effective; however, that the wet region on top of the block was only a minor elliptical spot and no oil flowed down the side boundary. The difference in the amount of oil collected at the bottom of the block and the amount of oil supplied at the top represents the cumulative drainage recovery from the block. Initially, the block did not contribute to the cumulative recovery, as the oil supply rate was equal to the single block gravity drainage rate. In Fig. 4 the cumulative recovery from blocks as a function of the dimensionless time is given. It is clear that the drainage of the upper block (oil supply) retarded the drainage of the lower block.
Fig. 4. Drainage performance of upper and lower blocks in a stack of two blocks.As shown in Fig. 4, up to tD = 0.08, there is no difference between the oil recovery from single block and two blocks. Later, the upper block produced almost twice as the lower one. One may therefore deduce that drainage of two blocks in a stack might take about twice the time for a single block. In Fig. 5, the single block behavior is compared to 2 blocks behavior. For the single block, the recovery is given as a function of dimensionless time. The time scale for the ‘two-blocks’ case is reduced by a factor 2, to take into account that drainage will take about twice the time of a single block.
Fig. 5. Comparison between the drainage performance of single block and a stack of two blocks.Indeed the drainage performance of the single block and the stack of two blocks seem to follow each other. If this trend also holds for a stack of more than 2 blocks, the drainage performance for a stack of n blocks (with equal properties), can be deduced from the single block drainage performance by adjusting the time scale by a factor n. For the actual reservoir this means that if it takes 1 year to recover a certain fraction of the oil from a block of 3 m height, it will take about 100 years to recover the same fraction of the oil from a 300-m stack of such blocks. Thus, it is important to determine the effective height of the stack of blocks in a reservoir. If no impermeable barriers of considerable lateral extent are present in the reservoir, the stack will have a height equal to the thickness of the formation. Only when permeability barriers are present, the stack can be divided into several sub-stacks, which would make the drainage process more favorable.
5.1. Oil addition to the top
To simulate the re-infiltration process when a gas cap is present inside the block, oil was added to the top surface of the block at a stepwise increasing rate. Initially, the block was at its gravity-capillary equilibrium and the oil saturation in the gas-invaded region was about 20%. The gas-invaded region itself had a length of about 34% of the block height. The average gas saturation of the block was equal to the ultimate drainage recovery of the block, being 27%. First oil was added at a rate of 3.7% of qmax to the top of the block. This oil was imbibed by the block without any liquid production from the bottom of the block. The average gas saturation of the block decreased to 21.7% before the first oil droplets appeared at the bottom of the block, and steady-state conditions were reached. With the assumption of constant oil saturation in the gas-invaded region, the estimated oil saturation increased from 20% to 36% during this time. After steady-state conditions had reached, the oil supply rate was increased to increasing values of q/qmax. The corresponding estimated average gas saturations are shown in Fig. 6 and Table 6. When the oil supply rate had reached 0.36 qmax, the wet region at the top of block covered the whole surface area indicating that we have reached the maximum gravity drainage rate at the presence of trapped gas. As expected, this rate is lower than the maximum single phase gravity drainage rate due to reduction in oil relative permeability. The trapped gas saturation in the gas-invaded region was estimated to be 35.5%.
Fig. 6. Average steady state gas saturation vs. oil flow.Table 6. Variation in saturation of the block after drainage with different oil supply rates.
| Rate of oil supply, percentage of q max | Average gas saturation of the block, % | Average oil saturation before steady state conditions, % |
|---|---|---|
| 3.7 | 21.7 | 36 |
| 7.4 | 18.7 | 44.5 |
| 14.8 | 15.3 | 54.5 |
| 29.6 | 12.5 | 62.5 |
5.2. Post gravity drainage
After the block had been allowed to drain again to its gravity-capillary equilibrium, the oil was added to the center of the (horizontal) top surface at a rate q = 0.36 qmax, being the maximum block infiltration rate in the presence of residual gas. The size of the wet region on top of the block was measured as a function of the amount of imbibed oil.
The results indicated that initially the size of the wet region was only 3.5% of the cross-sectional area of the block, but that the size gradually increased until the whole top surface was covered with a liquid film. At that moment oil production started at the bottom of the block and the average gas saturation of the block decreased from 27% to 12%. Both the size of the wet region and the average block gas saturation as a function of dimensionless time are presented in Fig. 7.
Fig. 7. Average gas saturation as function of dimensionless time during oil supply at the top of a block (q = 0.36qmax) and after drainage with q = 0.The small size of the initial wet region can be explained by the additional capillary pressure gradient in the transition zone between the oil-invaded top zone and the underlying gas zone. At the beginning, this transition was very sharp, so that both gravity and capillary forces contributed to the oil imbibition. When the oil/gas front moved downward, the capillary pressure gradient was smeared and gravity again became the dominating driving force for infiltration.
After steady-state conditions had been reached (oil production from the bottom equal to oil supply at the top), the oil supply was stopped. This allowed the block to drain the oil that had been absorbed during the re-infiltration process. The drainage performance of the block is also presented in Fig. 7. This drainage performance looks very similar to the single block drainage curve with an oil recovery of 12%. At a dimensionless time of tD = 2.9, we had recovered 80% of the amount that had infiltrated initially at a dimensionless time of 0.4. This is due to the fact that the production of oil started decreasing as the oil relative permeability in the gas-invaded region decreased. Consequently, the amount of oil absorbed during infiltration, was produced back afterwards but at a slower rate. The results of the imbibition experiments indicate that a considerable amount of oil had to be absorbed by the block before the relative permeability to oil in the gas-invaded region was high enough to allow oil to flow through this region. Hence, if the blocks within a stack restart draining due to some supplementary recovery process (e.g. reduction of the gas/oil interfacial tension), part of the oil produced from the upper blocks will initially be absorbed by the lower ones before the oil can be produced. This process seems to retard the recovery of the additional oil. The presence of a gascap region initially seems to be promoting the infiltration rate of the added oil to the top block, but, the absorbed oil will be produced slower later when the oil supply is stopped.
6. Imbibition and gravity drainage
In our third set of experiments, we investigated the drainage behavior under miscible conditions by submerging two oil saturated rectangular carbonate blocks in a solvent (see Fig. 8). These two blocks, had a permeability of about 2 Darcy and were laid on top of each other with a gap of 1.5 mm wide and a tilt angle of 20°. The height of the blocks was 5 cm, the length 10 cm and the width 3 cm.The blocks were filled with kerosene and the surrounding solvent was pentane .
Fig. 8. Experimental set up for the miscible gravity drainage tests.As soon as the blocks were submerged in the solvent, oil started to drain from both blocks into the fractures. To monitor this process, a drop of a colored solvent was added to the highest point of the fracture. After 10 seconds a colored mixture was observed at the lowest point of the fracture, indicating a flow rate of about 1 cm/s. As the total cross-sectional area of the fracture was 0.45 cm2, the total liquid flow rate leaving the fracture at the lower end was about 0.45 cc/sec.
For the non-tilted/non fractured blocks, using Eq. (1), the gravity drainage rate of the upper block was estimated to be 0.009 cc/sec. Notice that this rate is 50 times slower than the drainage rate from the upper block with the flow rate through the tilted fracture. i.e., oil flow constitutes only 2% of the outflow mixture with the balance being solvent. This fast convection current seems to wash away the oil leaving the upper block, thus, preventing this oil from infiltrating the lower block. However, the lower block was draining as well, imbibing the mixture passing through the fracture. But, the imbibed mixture is mostly solvent with negligible oil. This implies that the blocks are draining almost independently. This behavior is completely different from the gas/oil drainage behavior, where oil leaving the upper block would completely imbibe into the lower block.
The flow rate in the tilted fracture was estimated from:(4)where:
-
d = fracture width (=1.5 mm)
-
= tilt angle (=20°)
-
S = cross-sectional area of fracture (=0.45 cm2)
As the mixture contained only about 2% oil, the difference in density between the mixture and the surrounding pure solvent was only 0.2 × 0.15 = 0.003 g/cm3, and the viscosity of the mixture was close to the solvent viscosity (0.2 CP). Thus, qmix≈0.4 cm3/s.
This value agrees well with the observed rate of 0.45 cm3/s i.e., the high convection current may be explained by the high fracture permeability.
7. Miscible/immiscible gas injection and gravity drainage
Two additional tests were carried out to investigate immiscible and miscible gravity drainage in our fourth series of experiments. These were:
-
-
Injection of both equilibrated and enriched gases into a single block carbonate rock (with open block sides)
-
-
Injection into a stack of four carbonate blocks (with all sides open).