Experimental and numerical approaches to investigate the out-of-plane response of unreinforced masonry walls subjected to free far-field blasts

1. Introduction

Research on blast events has increased vastly in the last years, due to the observed increment of threats against buildings, monuments and infrastructures in populated areas. Such threats can come in the form of vehicle-borne improvised explosive devices (car bombs) and person-borne improvised explosive devices, or a combination of these. The extent of damage which can be induced by these threats is highly dependent on the explosive charge and stand-off distance from the building. Incidents that have occurred recently are, for example, the bomb explosion in the government quarters in Oslo on July 2011, and the 2017′s semi-trailer truck attack in the city centre of Stockholm.

Masonry structures are very common in the existing international building stock, especially in the form of perimetral walls [1]. In the event of blasts, unreinforced masonry (URM) walls may be subjected to very high pressures. Masonry has limited capacity against out-of-plane actions, particularly when unreinforced, as a result of the reduced tensile strength of the mortar and the brick–mortar interface [2], which lead the walls to out-of-plane failure mechanisms. It is common that explosions take place at the ground level of a building. The out-of-plane failure of the perimetral walls can induce flying debris inside the building and, in low- to medium-rise buildings, may affect the overall stability of the construction [3].

The permissible deflection of the walls must be limited in order to reduce structural damage and casualties. The prediction of the out-of-plane response of URM walls to shockwaves proves, nonetheless, very challenging. In general, when subjected to out-of-plane dynamic loads, URM walls experience bending and rocking [4], [5], [6]. The bending/rocking response is highly non-linear as it depends on the wall’s geometry, masonry properties, as well as boundary and loading conditions [7], [8], [9], [10], [11], [12]. Under blast loads, other forms of out-of-plane failure mechanisms can be activated, like shear failure at supports [14] and, in some circumstances, uplifting [6]. The wall’s response becomes related to the parameters of the blast action, that is, the stand-off distance and the weight of the explosive, as well as the presence of reflection, diffraction and clearing effects that the shockwave runs into along his path [15], [16], [17]. As a result, a description of the wall’s response capable to embrace multiple blast scenarios becomes difficult, and accurate insights seem achievable primarily through experimental and numerical tests carried out on a case-by-case basis.

In this paper, state-of-the-art test methods and modelling approaches pertaining to the investigation of URM walls subjected to blasts are reviewed. Interest in the experimental and numerical response of masonry structures to blasts has been rather scarce in the literature, see e.g. [18], being mainly devoted to the response to quasi-static and seismic loads [19]. A large increase in interest towards blast loading has however been noted in the last two decades [3], [20], [21], [22]. The review contained in this paper covers methods and approaches used for the investigation of the dynamic response of masonry structures in the event of moderate- to far-field, free-field blasts. Attention is given to regular structures and, more particularly, to load-bearing masonry walls with no openings, which are usually found in commercial and residential buildings. The focus is on recent tests and models investigating the lateral flexural/torsional capacity of masonry walls, also referred to as out-of-plane capacity in this manuscript, to blast loading.

The review is based on the publicly available literature, i.e. on non-confidential documents. The review of the test methods is systematic, as it identifies common themes among the suitable literature. Key themes include the preparation of the test items and test setups, boundary conditions tested, measurement techniques utilized, and failure mechanisms observed. A methodological review is also performed on the most recent modelling approaches. Models for URM walls are divided into classes depending on the level of detail reached, and their performance is evaluated based on cases reported from the literature. An overview of models for the blast and wall-blast interaction are included in the review.

Masonry structures of curved geometry, like arches, vaults, and domes, such as those discussed in [24], are not included in this review. This review also excludes strengthening and retrofitting techniques designed to protect URM walls from the effects of blasts, as this topic was reported in previous works [25], [26], [27], [28]. For a more comprehensive description of models for the blast and wall-blast interaction, we refer to [23]. Finally, the reviews carried out in [28], [29] contain detailed expressions for empirical models approximating the blast action, which is not treated in this survey.

2. Categories of out-of-plane actions

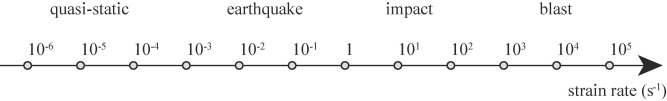
URM walls have a very low flexural and torsional capacity as compared to other construction materials commonly used in the field of civil engineering, mainly because of the weak tensile strength of the mortar and the brick–mortar interface. This results in very high vulnerability to lateral, out-of-plane loads, i.e. actions that act perpendicularly to the wall’s mid-plane. Different categories of out-of-plane loads can be distinguished, depending on the loading rate involved and the strain rate activated in the material (Fig. 1):

1.
Quasi-static loads, such as those due to the self-weight of the structure, or alternatively, due to reactions coming from adjacent structural elements like return-walls [30], or engendered by eccentricities of the vertical loads applied at the top of the wall [31], [32], [33]. This category includes pressure exerted by the action of wind, water, snow, and soil onto the wall surface, when the latter can be modelled as sources of action with little time variation. Additional sources of quasi-static loads are settlements [35], [144], [145], and creep [36], generating long-term effects on the structure.
2.
Slow dynamic loads produced by seismic events, that is, gravity loads engendered by the relatively high mass-density of the bricks, or by other structural elements connected to the walls, such as massive floor slabs, or high-span roof structures [11], [12].
3.
Fast dynamic loads generated by impacts, i.e., by bodies travelling and colliding with the wall at high speed [38], [114], [141], [142].
4.
Dynamic loads generated by blasts, which is the focus of this paper.

In the event of a blast, shockwaves are generated. With respect to categories 1–2 listed above, the shockwaves involved in an explosion are characterized by a very fast increase of energy followed by a slower, but still fast, release of energy. While for ordinary quasi-static loads (category 1) the strain rate is in the range of 10-6-10-4 s−1, loads generated by impacts (category 3) and blasts (category 4) yield a strain rate ranging from 10 to 105 s−1 in the structure, which is hundreds to thousands of times higher than the one generated by earthquakes (category 2) [39]. The strain rate resulting from blasts depends especially on its distance from the wall and on its charge. At such high strain rates, magnification of the masonry properties, notably stiffness and strength, occurs [40], [41], [42], [43].

3. Classes of blast events

Depending on the distance at which the explosion is vented, its effect on the structure can be different. In the literature, three classes of blast events are classically distinguished (Fig. 2):

1.
Class 1 - Moderate to far-field blasts: When the explosion is far enough from the wall such that shock waves strike the wall face as a pressure load (Fig. 2a). Due to the relatively far distance of the explosion from the target, the blast wave impinges the target instantaneously with an almost uniform pressure [3], [44], [45].
2.
Class 2 - Near-field or close-in blasts: When the explosion is close enough to the structure not only to produce air shockwaves, but also to induce ground shakes (Fig. 2b). These ground shakes are characterized by a very short period, and by the presence of both horizontal and vertical components [39], [40], [46], [47]. Near-field blasts generate shockwaves that depend on the directionality of the charge and are therefore likely to strike the wall surface in non-uniform way [48]. Ground reflections and clearing effects of the shockwave are also more pronounced in near-field blasts [16].
3.
Class 3 - Contact or embedded blasts: When the explosion occurs on the surface the wall or partially within the wall (Fig. 2c). This class involves dynamic loads belonging to the third and fourth categories of out-of-plane actions listed in Section 2. In fact, a fraction of energy is transmitted to the wall, and propagated inside it in the form of stress waves. As in an impact, the induced stress waves can cause extreme material compaction, which can lead to the compressive failure of masonry, up to complete disintegration and loss of strength, which, upon unloading, causes cratering in the proximity of the charge [3], [49].

For the three mentioned classes of blast events, debris may separate from the main body of the wall and travel from it at high velocities. This occurs especially during blast events of class 3, when the compressive stress waves reach the backside of a wall, and are reflected as tensile stress waves, making high amounts of small-size debris flying apart from the wall [49]. Debris of larger size form in blast events of class 1 and 2, because of the large horizontal velocity component experienced by the wall during the formation of the failure mechanism [50], [51], [52]. Despite their size, flying debris establish an additional source of load and danger to people and structures located within a certain distance from the wall [14], [21], [50], [53].

Regarding the position of the explosion with respect to the building, distinction is made between free-field blasts [3], [45], and internal, or in-closed blasts [21], [54], [55]. In this review, we focus on actions produced by free-field, far-field blasts. Blasts belonging to this specific class produce on the wall surface an overpressure-time diagram that is typically characterized by a phase of positive pressure, followed by a slower phase of negative pressure (Fig. 3a). During internal blasts, more complex diffraction and reflection phenomena occur, so that the structure enclosing the explosion is subjected repetitively to an overpressure whose peak value progressively decreases over time (Fig. 3b), see e.g [55]. Moreover, in internal blasts, non-planar blast waves impact the walls, as opposed to the planar blast waves usually generated by free far-field explosions; as such, the charges cannot be idealized as hemispherical or spherical ones [54]. Internal blast pressures affect the exposed structure, by weakening its corner, or lateral restraints [21], but also by inducing the uplift of the ground-floor ceiling [54]. As observed in studies on the seismic performance of masonry buildings [56], [57], [58], [59], this uplift dramatically reduces the level of constraint of the wall, making it extremely vulnerable to out-of-plane actions.

4. Test methods

In this section, state-of-the-art test methods for URM walls subjected to free far-field blasts are reviewed (4.1 Test objects, 4.6 Observed failure mechanisms). The review aims systematically to explore different aspects of the test methods, which are the test objects, test setups, boundary conditions, measurement techniques, test designs and observed failure mechanisms. Test methods for the out-of-plane static behaviour of the walls, known as pushover tests, are included in the survey (Section 4.7), as they are proven to be a simple and effective tool used in the field of blast engineering for the preliminary investigation of the wall resistance function, and for the validation of models prior to their extension to blast loads, see e.g. [61].

4.1. Test objects

Most of the tests reproducing far-field blasts were carried out on full-scale URM walls that are representative of the construction practice, i.e. with the height of one storey (2–3 m), and with the width of a panel spanning between two openings (1–3 m) [42], [45], [61]. The majority of the walls tested were built with building blocks made of solid clay bricks [3], [21], [42], [47], [62], [63], and hollow concrete blocks [20], [42], [44], [45], [61], [64]. Only few tests were carried out on modern hollow clay-brick walls [22], [42]. For all these types of blocks, cement-mortared joints were used.

Tests on scaled walls were much less frequent than those on full-scale ones. Dennis et al. [52] and Baylot et al. [51] performed tests on ¼-scale fully, partially and non-grouted hollow concrete-block walls. The scaling laws used in those studies were shown in [51]. Alsayed et al. [64] performed tests on ½-scale non-grouted infill concrete-block walls built in scaled RC frames.

The test items were tested as is, or, alternatively, after being prepared in a more specific manner to support test observations. Pereira et al. [61] drew a regular mesh using black tape to better visualize the movement of the wall surface. Keys and Clubley [21], [65] and Baylot et al. [51], see Eamon et al. [14], painted the masonry panels with different colours to distinguish the individual sections of the test object (Fig. 4). To help analyse the debris mass distribution, in [21], [65] every brick was assigned a unique number, and a grid was marked on the ground.

4.2. Test design and pilot tests

Given the complexity of a blast test, preliminary numerical simulations are usually carried out to design the test and verify the test setup and blast parameters. Simplified blast load distributions [52], pressure-impulse diagrams [45], and numerical models for the blast action can be used for this purpose. The Conventional Weapon (ConWep) model contained in the TM5-855 US army handbook, in both its variant for finite and infinite reflection surfaces, is often used due to its simplicity to estimate reference parameters of the blast load, like the total duration, positive and negative phases duration, impulse, and equivalent stand-off distance, see e.g. [64].

The use of pilot tests to be carried out on ‘control walls’ prior to the blast tests proves very helpful for checking the effect of the test setup, and the variation of the wall properties throughout the experimental campaign. Beak et al. [63] performed a static test on a URM wall up to wall failure and compared the static and dynamic load-bearing capacities of the wall. Moreover, before any blast test was performed, a forced-vibration test and an impact test were carried out to establish the change in fundamental frequency, modal shapes, damping coefficient, and wall stiffness with the increase of impulse. Pushover tests were also performed by Dennis et al. [52] and Baylot et al. [51] prior to the dynamic tests, in order to determine the force–displacement relation for the walls.

4.3. Test setup

In moderate- to far-field blast events, the wall is not put in direct contact with the charge, but it is exposed to the blast wave produced by it (Fig. 5). A blast wave can be triggered in different ways. Free far-field explosions can be engendered by using certain amounts of explosive, placed at various stand-off distances from the target. TNT was often used [3], [44], [62]. The bottom of the charge was placed at the same height as the bottom of the wall [52], or at least 0.3–1 m above the ground [42], [54], [62]. When the explosion was triggered at a low-to-moderate distance from the wall, the charge was placed at a certain height from the ground, in order to prevent possible ground shocks [64]. For small stand-off distances, the height of the charge was increased to obtain a uniform pressure distribution over the specimen [42]. In fact, in near-field explosions, the shape of the charge and the central point of ignition may cause significantly different scenarios [40]. As an alternative to TNT, far-field explosions were generated by using a surface burst ammonium nitrate/fuel oil (ANFO) charge [45].

When testing single structural elements to far-field blasts, a source of issue is given by the blast-wave clearing effect [16], [65]. This phenomenon is usually accompanied by a sudden drop of pressure, which can finally lead to a high level of uncertainty regarding the blast wave parameters to be reproduced in the test [45]. In Dennis et al. [52], the reaction structure, i.e. the walls located above and at the sides of the test items, were extended to prevent relief of the blast pressure around the test item. A similar setup was used by Gagnet et al. [20] to represent the remainder of a larger structure in a real situation. For the same purpose, steel containers were used to house the walls in [45].

A similar issue is given by the wraparound effect, which occurs when part of the blast wave propagates from the front to the rear of the test item, passing around its edges and resulting in increased pressure at the rear [45], [65]. In [52], a tunnel surrounding the specimen was added to ensure that the blast wave wrapping around the test item would not influence the results.

In some studies, a reinforced-concrete chamber was built to contain vented gas explosions impacting the wall placed at one of the chamber ends [22]. Beak et al. [63] ignited methane-air mixture to generate blasts inside the chamber; different gas overpressures were obtained by changing the gas volume, vent area and methane concentration. The use of a testing chamber proves a promising space-saving solution. Another solution to be taken when the explosion takes place inside the testing facility consists in fitting the testing room, at its exhaust, with a rarefaction wave eliminator, in order to reduce unwanted reflections and complex airflow interfering with the specimens [21]. Finally, Pereira et al. [61] used confined underwater blast wave generators to produce distributed shockwaves on the wall surface. Thanks to the higher density of the water, the liquid containers were placed in direct contact with the wall, lowering the charge necessary to trigger the explosion, and limiting the amount of debris, pollution and noise in the test bed.

4.4. Measurements

Blast tests pose several challenges to the measurements during testing, primarily due to a very high loading rate, high temperature and sudden shockwave. Dealing with explosions, it is important that the data acquisition system is placed far enough from the specimen to avoid signal distortion [45], [67], and protect it from heat waves [42]. Measurement devices that have typically been applied for dynamic testing in literature, such as displacement and pressure transducers, accelerometers, strain gauges and high-speed cameras, are mentioned herein. An example of instrumentation plan displaying the measurement used in a blast test is given in Fig. 6.

Displacement transducers were fixed on the non-exposed wall face to obtain displacement time-histories at different points of the URM walls [3], [22], [42], [45], [63]. Alternatively to displacement transducers, laser deflection gauges were used [44], [61].

The wall acceleration is considered an important element in the response of the wall to blast loads [44], [51], [52]. The velocity and the displacement time-histories can be derived from the acceleration. When facing explosions, URM walls experience failure-mechanisms with a dominant horizontal velocity component, which appears to depend on the impulse applied to the wall [51]. The vertical component, along with the wall rotation, can be, nonetheless, large; for this purpose, in some tests, multi-axial accelerometers were installed on the wall face [52], [62].

Another feature which could be measured is the local deformations of the wall. Deformations were measured at specific wall locations by means of strain gauges [62]. From the strain measures, the principal stresses were derived and used as justification for the observed failure patterns [62].

Pressure transducers are the most common measurement device. They have been used to measure the pressure history in the close surroundings of the wall face exposed to the blast loading [20], [22], [45], [51], [52], [61], [63], [64]. The tests were instrumented with pressure transducers monitoring both the static and the dynamic pressure [21]. In the case of an explosion taking place in a pressure chamber, the pressure recorded in the testing chamber is seemingly the same everywhere, because wave pressures travel at the speed of sound [22]. In the case of free far-field explosions, pressure sensors were installed on the stiff frame surrounding the wall [45], [67]. Gauges were also installed to measure the free-field pressure at the same stand-off distance than the walls [44].

High-speed cameras are a common tool in blast tests, as they allow the failure mechanism to be recorded and therefore observed and analysed even after the blast event [21], [22], [44], [45], [51], [52], [61], [62], [63]. In [68], high-speed cameras were used to track the displacement of the wall at various marked points. In other tests, they were used to observe the size of the breach created by the explosion and the amount of wall thickness removed in the form of debris [3]. From high speed cameras, the maximum velocity of the travelling debris was also measured [3]. Hazard levels for building occupancy can be defined based on different levels of the debris horizontal velocity [45], [51].

4.5. Boundary conditions

URM walls were tested to blast events under boundary conditions that were representative of common construction practices. Achieving realistic boundary conditions for wall specimens is nonetheless challenging in any experiment. Cantilever walls were tested by Ahmad et al. [62] and Keys and Clubley [21]. Michaloudis and Gebbeken [3] constrained the walls into a stiff frame. No construction details were provided [3], and the walls were modelled as simply supported at their four extremities in subsequent numerical simulations [24]. Boundary conditions activating arching actions inside the walls were explored by Gagnet et al. [20]. However, post-blast inspections revealed that the corners of the support framing around the walls were cracked, indicating that the supports did not provide the intended action [20]. Beak et al. [63] tested panels that were clamped on all sides. Three types of bonding between the reinforced-concrete (RC) frame and the brick work were constructed by Varma et al. [47], namely dowel pins, grooved and plain bonding. From the test results, it appears that the different types of bond configurations produce simply supported boundary conditions on all four sides.

The failure mechanisms experienced by the wall depend on the boundary conditions. Different failure mechanisms were achieved by placing the walls into a RC frame and ensuring displacements on the vertical sides of the walls by putting insulating foam [44] or by leaving an air gap between the wall and frame [51]. Boundary conditions allowing both one-way and two-way bending failure-mechanisms were also tested by Li et al. [22]. Tests on boundary conditions ensuring one-way, vertical bending failure-mechanisms of the walls were found in many experimental campaigns, see e.g. [44], [45], [51], [52]. These simplified boundary conditions are not only encountered in real buildings [69], but are also used as a preliminary step towards the investigation of more complex wall configurations by means of numerical models [70].

4.6. Observed failure mechanisms

When exposed to far-field blasts, URM walls typically exhibited an out-of-plane failure mechanism that was mainly characterized by the development of cracks within the mortar joints, while negligible damage was observed in the bricks [3], [45], [65], Fig. 7. More specifically, for walls failing under a one-way vertical bending mechanism, a horizontal crack formed over the entire length above the wall mid-height, followed by the rocking of the two separated regions [44], [45], [51]. In cantilever walls, a similar crack formed above the wall base [21], [62]. For walls failing under a two-way horizontal-vertical bending mechanism, the walls crack according to a pattern resembling to that predicted by the yield-line theory [22], [63].

4.7. Quasi-static test methods

Pushover tests consist in applying a load to the wall whose direction is perpendicular to the wall’s mid-plane, and whose magnitude increases slowly and incrementally. The load normally consists of a force or pressure. The former can be applied through hydraulic jacks and redistributed on the wall face by a system of beams [42], [71], [72], [73], [74]. The latter can be applied either by air- or water-bags, disposed along lines [75] or over the entire wall face [76], [77], [78], or by a chamber with pressurized water [52].

Quasi-static tests yield the resistance function, i.e. the diagram relating the resultant force to the displacement of a control point of the wall. Applying the load by controlling the displacement enables the derivation of the post-peak branch of the resistance function [79]. More generally, performing pushover tests gives insights on the most important features affecting the wall behaviour, namely: the presence of openings [80], boundary conditions and test setup [72], presence of return walls perpendicular to the wall [81], effect of confinement, i.e. reinforced-concrete fames surrounding the wall [82], application of strengthening techniques [83], level of applied vertical load [76], aspect ratio of the wall [78], anisotropic property of the masonry given by the orientation of the mortar joints inside the wall [75], [77]. The stiffness, force and displacement capacity of the wall that are derived from these tests [84], [85], [86] can be compared to those obtained from blast tests, to establish useful relationships between the observed wall’s static behaviour and dynamic response.

5. Numerical models

The numerical modelling of blasts and their effect on URM walls is challenging, as it requires techniques capable of handling the fluid-wall interaction, the increase of strength and stiffness in the masonry materials due to high strain rates, and the highly non-linear dynamic response of the walls, which, in close-to-collapse situations, may experience large displacements and rotations, along with fast contact closure/separations. This section reviews modelling approaches used so far for the response of URM walls (Section 5.1) and blast actions (Section 5.2). The approaches are general and therefore applicable not only to regular structures like walls, which is the focus of this paper, but also to masonry structures with more complex geometry.

5.1. Modelling of the URM walls

Masonry is a composite material made of bricks and mortar. Due to its composition, it is heterogeneous, and anisotropic. It exhibits a non-linear material behaviour in the bricks, mortar, and brick–mortar interface, which evolves at high strain rates [40], [61], [87]. Moreover, the different sizes and shapes of the bricks, along with the different brickworks, confers scale parameters to the material which are essential to model [88], [89], [90], [91]. The strategies that are classically used for modelling both the static and the dynamic behaviour of URM walls are illustrated in Fig. 8. Distinction is made between [92], [93], [94]: macro-, or macro-scale, models; simplified micro-models, also known as meso-scale models; detailed micro-, or micro-scale, models. For a more comprehensive survey, we refer to [93], [95]. The focus of the present review is on the numerical models dedicated to URM walls subjected to blasts.

5.1.1. Macro-models

Macro-models are models where bricks and mortar are smeared into an equivalent orthotropic continuum and their interaction is invoked by means of a homogenous constitutive law (Fig. 8). The equivalent properties of the continuum can be derived either by using ad-hoc assumption taken for the material [2], [74], [85], [96], [97], [98], [99] or by the use of homogenization [100], [101], [102], [103], [104] and multiscale techniques [105], [106], [107], [109].

The use of macro-models does not provide information on the local behaviour between the masonry components, but yields a reliable prediction of the overall masonry behaviour for a much more reasonable computational cost [46], [110]. For this reason, there are many examples of macro-models in the framework of blast simulations (Fig. 9). Wei and Hao [111] derived a continuum damage model for masonry including strain-rate effects, based on a 3D numerical homogenization method. Wong and Karamanoglu [112] modelled the wall as a homogeneous solid material with isotropic elastic properties and using as failure criterion a generalization of the Drucker-Prager criterion. A similar model was employed by in [61] to reproduce the results of blasts tests and complement them by means of parametric studies. The influence of the strain rate on the material properties was introduced by a dynamic increase factor (DIF) obtained after calibration with the test results. Wang et al. [53] modelled masonry by means of a homogenized constitutive law based on the work of Wei and Hao [111], including strain-rate effects. Parisi et al. [69] modelled masonry as a no-tension material with limited strength in compression. Rafsanjani et al. [113] developed a strain-rate dependent anisotropic continuum model for masonry similar to the one of Lourenco [2]. Alsayed et al. [64] used an equivalent homogenized material model for representing masonry infill walls in RC frames. Silva et al. [114] used a rigid block-spring model with masonry properties derived from homogenized stress–strain relationships obtained by through-the-thickness integration techniques made for plates. Strain-rate effects were included by means of a DIF. In [55], the structure of Rome’s Pantheon was modelled under blast by assuming the various layers of granite, bricks, mortar and roman concrete that compose the structure as made of homogenous materials described by linear model in compression and a smeared constant crack model in tension.

5.1.2. Simplified micro-models

Simplified micro-models are idealizations in which the mortar and the brick–mortar interfaces are represented by joints of equivalent composite properties, and the blocks are expanded to cover the joint thickness (Fig. 8). In some cases, a vertical joint is introduced in the blocks to simulate the block splitting. To this class belong finite element models making use of interface elements, e.g. [115], [116], discrete or finite/discrete element models based on contact formulations [117], [118], [119], [120], [121], applied element models [122], [123], [125], and rigid block models [126], [128], [143].

Only few examples of simplified micro-models for blast simulations can be found in the literature, some of which are illustrated in Fig. 10. Keys and Clubley [65] developed an applied element model to reproduce the results of far-field blasts on cantilever masonry walls. Michaloudis and Gebbeken [3] developed a finite element model for far-field and contact blasts, using a specific model for strain-rate effects for bricks, and a 3D interface mixed-mode delamination model for interfaces. Eamon et al. [14] modelled the cross section of vertically-spanning walls as a single width column of vertically-stacked blocks, including strain-rate effects. For representing crushing, elements were deleted from the model when the compressive stress was reached. Masi et al. [24] simulated URM walls and curved structures subjected to blast loads by means of the discrete element method. Rigid block models for blast simulations of free-standing walls and museum artefacts were formulated and used in [6], [146].

5.1.3. Detailed micro-models

Detailed micro-models consist in modelling the bricks and the mortar joints with their real size and thickness [19], [130]. At the highest level of detail, interface elements can be used to describe the debonding at the brick/mortar interface [19], as well as the cracking within the mortar layers [131], [132]. Through this modelling technique, the composite action between bricks and mortar as well as the various local failure modes occurring within the two materials are represented in realistic manner.

Only few examples of detailed micro-models can be found in the literature dedicated to blast simulations (Fig. 11), mainly because of the extremely high computational burden that this modelling approach requires combined with the already high demand required to run blast simulations. Sielicki and Łodygowski [40] developed a detailed micro-model of a concrete masonry wall. The concrete and the mortar were modelled by a user-defined material calibrated on material tests investigating the strain-rate effects on the material properties. The model developed by Wei and Stewart [133] was based on the work of Wei and Hao [111], where a modified Drucker-Prager model adapted to include strain-rate effects based on the results of dynamic uniaxial compressive tests [134], was used for a user-defined material for bricks and mortar. Li et al. [22] modelled the bricks and mortar with an in-software material law, by increasing the compressive and tensile strength by a DIF to model strain-rate effects.

5.1.4. Comparison between models

Table 1 shows advantages and drawbacks of the classes of numerical models discussed in the sections above. The main advantage of using macro-models is to significantly limit the computational cost, allowing numerical studies to be carried out on larger structures. Wang et al [53] showed that, dealing with blast simulations, the calculation time of a macro-model can be up to 60 times lower as compared to a detailed micro-model. The decrease in cost is mainly related to the number of elements to be used in these models: several hundred versus several thousand or millions.

Table 1. Comparison of modelling approaches for masonry walls.

Class	Advantage	Shortcoming
Macro-models	Low cost	Calibration on structural tests
Simplified micro-models	Calibration on material tests	High cost
Detailed micro-models	Calibration on material tests	Prohibitive cost

Besides the number of elements, the cost is also controlled by the minimum element size. In general, the results of blast simulations are highly sensitive to the element size [40], which should be determined based on the loading duration and the stress-wave speed [111]. In detailed micro-models, the size of the elements assigned to the mortar is smaller than those assigned to the building blocks [53]. Moreover, while under static or slow dynamic loads, such as those experienced in seismic events, the damage of the out-of-plane loaded masonry is dominated by the cracking of the mortar and brick–mortar interface, under very high strain rates the walls may exhibit crushing and fragmentation in the bricks [3]. The modelling of these phenomena becomes important when debris simulation is aimed. Lagrangian approaches based on the finite or discrete element method can be used to tackle this issue, see e.g. [3], [53], which further extends the cost.

The minimum element size is directly related to the calculation time-step when explicit (forward-Euler) solvers are used [53], [135]. Solvers of this type are mostly used when blasts are modelled. The use of short time-steps guarantees numerical stability in explicit procedures and fits well with the very high loading rates engendered during blasts (Fig. 1). To increase the time step, implicit (backward-Euler) calculations can be attempted, both for micro- and for macro-models [114], [136], even though careful evaluation is needed based on the model complexity.

Simplified micro-models limit the computational cost compared to their detailed counterparts, especially when building blocks are modelled as rigid bodies [24]. Macorini and Izzuddin [136] showed that the computational cost of simplified micro-models can be speed up by 16 times when domain decomposition techniques are employed. To reduce the computational cost of a macro-model, Masi et al. [55] employed parallel computing to divide the geometric domain into several subdomains to be solved separately.

The increase in stiffness and strength due to high strain rates poses an issue on correctly reproducing the response of URM walls to blast. Available studies show that its strength can be amplified by up to 6 times in compression and 10 times in tension [40], [134], see also [133]. In most of the models reported in this review the influence of the strain rate on the masonry properties is included by means of a DIF, which does not vary with the strain rate. In that perspective, the main advantage of micro-models, both detailed and simplified, appears to be the possibility of having their calibration based on the results of material tests, and using structural tests on masonry components only as a final benchmark (Table 1). Material tests are more affordable and can be repeated to diminish the uncertainty interval on the variation of the material parameters versus the stain rate. Furthermore, through micro-models, one can model the effect of the brickwork and boundary conditions in a more realistic way, which poses a challenge to macro-models.

5.2. Modelling of the blast action

There are several strategies that can be used to model the load exerted by a shockwave on a structure. The most popular ones are illustrated in Fig. 12 for in-closed blasts and reported in this section. The classification follows the one proposed by [23]. The modelling approaches herein described differ in their ability of modelling interactions, reflections, and in the related computational cost, see Table 2.