A Breakthrough in Pressure Generation by a Kawai-Type Multi-Anvil Apparatus with Tungsten Carbide Anvils

1. Introduction

A multi-anvil press is a static high-pressure apparatus in which a sample is compressed with more than three anvils. The Kawai-type multi-anvil press (KMAP) is one of the most widely used high-pressure apparatuses in the field of geophysics [1]. A main feature of the KMAP is its double-stage compression system, in which pressure is generated by squeezing an octahedral pressure medium by the truncated corners of eight cubic second-stage anvils that are synchronously compressed by six first-stage anvils. The main advantages of the KMAP, in comparison with the other widely used high-pressure apparatus, the diamond anvil cell (DAC), are its much larger sample volumes, more stable temperature over time, and more homogeneous pressure–temperature fields. For example, KMAPs have sample volumes that are three orders of magnitude larger than DACs in the same pressure range, and higher precision by one order of magnitude in pressure and temperature control. These advantages allow the KMAP to yield highly reliable experimental results; therefore, KMAPs have been particularly utilized for the precise determination of the phase relations and high-temperature physical properties of mantle minerals. Recently, KMAPs have also been used in the field of materials science, for the syntheses of novel materials at pressures that cannot be reached by any other large-volume presses [2].

One reason for the wide use of KMAPs in studies of the Earth’s interior is that they routinely allow pressures of up to 25 GPa to be generated [3]. Various phase changes in mantle rock-forming minerals occur in this pressure range, which corresponds to depths as low as the uppermost lower mantle in the Earth’s interior (∼700 km). It was considered for many years that the composition in the deeper parts of the lower mantle is homogeneous, and that no remarkable changes are likely to occur in the phase stabilities and physical properties of the minerals there. Therefore, pressure generation of up to 25 GPa has been sufficient for many researchers.

Recent seismological studies, however, have shown slabs subducting into the lower mantle and settling at a depth of around 1000 km (∼40 GPa) [4]. Another study has shown that although mantle plumes from the core-mantle boundary are vertically straight to a depth of 1000 km, they bend in shallower regions [5]. Thus, it is desirable for KMAPs to be developed such that they can be used to expand the exploration of the chemistry and physics of mantle minerals to pressures greater than 40 GPa.

Improvement of KMAP technology in recent years has enabled pressures of 60 GPa to be reached by utilizing sintered diamond (SD) second-stage anvils [6], [7]. The latest technology of SD anvils makes it possible to generate pressures over 100 GPa [8], [9]. However, the cost of SD anvils is much higher than that of tungsten carbide (WC) anvils. Special techniques for manufacturing anvils and high-pressure cell parts in combination with a precisely aligned guide-block system are also necessary for successful high-pressure and high-temperature generation. Moreover, the edge lengths of SD anvil cubes are 10–14 mm—less than half that of WC anvils (26–32 mm). Therefore, research groups that can practically conduct experiments using SD anvils are limited. In contrast, WC second-stage anvils have been widely utilized in KMAPs because they are less expensive and more tractable than SD anvils. For these reasons, we have made improvements in a KMAP with WC anvils to allow it to generate pressures greater than 40 GPa [10], [11]. Other researchers have recently developed KMAP technology with WC anvils that can achieve pressures of up to 50 GPa at room temperature [12]; however, our particular focus is on high-pressure generation under high temperatures.

This paper reviews our recently developed methods for pressure generation up to 65 GPa, which greatly exceeds the conventional limitation of pressure generation in KMAPs with WC anvils. In this paper, we refer to pressures greater than 40 GPa as “ultrahigh pressures.”

2. Methods

2.1. Guide-block system

The first- and second-stage anvils compress cubic and octahedral spaces, respectively. High pressures are sealed by gaskets made of pyrophyllite. Ideally, the thicknesses of the gaskets must be identical for ultrahigh-pressure generation, because any difference in the thickness weakens the high-pressure sealing by the gaskets. For this purpose, the cubic and octahedral compression spaces must be highly symmetrical.

Conventional KMAPs employ first-stage anvils and a guide-block system, in which a second-stage anvil assembly is vertically oriented in the [111] direction [13], [14], [15] by placing two sets of three first-stage anvils on the upper and lower sides. Although six first-stage anvils are simply placed in a guide cylinder in the Walker module, two sets of three first-stage anvils are fixed in the upper and lower guide blocks in other types of KMAP. These two sets of first-stage anvils are driven by a uniaxial press. Applied press loads in these configurations, however, tend to distort the cubic compression space rhombohedrally and cause blow-out when attempting to generate ultrahigh pressures.

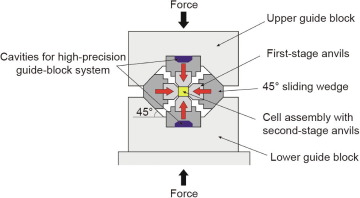
In order to prevent rhombohedral distortion, we have employed the Osugi-type guide-block system [16], which is also known as the DIA-type system† in the high-pressure science community (Fig. 1). The Osugi-type system consists of upper and lower guide blocks with four 45° slopes, on which four sliding wedges are located. Each of the guide blocks and sliding wedges is equipped with a first-stage anvil. The uniaxial force causes the four wedges to advance, so the cubic space is synchronously compressed by the six first-stage anvils in the [100] directions. This configuration causes no rhombohedral distortion.

The principal issue with the Osugi-type system is that the cubic compression space is tetragonally distorted by the application of press loads, due to the difference in supporting strength for the first-stage anvils between the guide blocks and the sliding wedges. The distortion of the cubic space varies vertically and horizontally as the press load increases. This causes a difference in the vertical and horizontal gasket thickness between the anvil gaps, and increases the frequency of blow-out. To solve this issue, the supporting strengths for the upper and lower first-stage anvils were adjusted by manufacturing cavities in the guide blocks (Fig. 1). As a result, the dimension of the compression space in the horizontal and vertical directions remains essentially identical under any press load. We hereafter refer to the Osugi-type guide-block system with this improvement as the “high-precision guide-block system.”

To date, three KMAPs with the high-precision guide-block system have been built in the world: The first is SPEED-Mk.II in the BL04B1 beamline at the synchrotron radiation facility of SPring-8, Japan [17]; the second is MADONNA-1500 at the Geodynamic Research Center at Ehime University, Japan [18]; and the third is IRIS-15 at the Bayerisches Geoinstitut University of Bayreuth, Germany [10]. Other KMAPs have also been constructed with the Osugi-type guide-block system, but the anvil-supporting strengths of these systems have not been adjusted. One example of the latter is SPEED-1500, which is also in the BL04B1 beamline at the SPring-8 synchrotron radiation facility. The horizontal dimensions of the cubic compression space in SPEED-1500 increase relative to the vertical dimensions against a press load (+13 µm·MN−1 [17]). In contrast, this rate was suppressed to +4, +1.6, and –0.07 µm·MN−1 in the cases of SPEED-Mk.II, MADONNA-1500, and IRIS-15, respectively. Note that the guide blocks of SPEED-Mk.II were replaced by those of MADONNA-1500, and SPEED-Mk.II is now called SPEED-Mk.II-D. The highest pressure generated by SPEED-1500 using SD anvils is 44 GPa [19], whereas a pressure of 120 GPa has been generated by SPEED-Mk.II-D [8], [9], clearly demonstrating the usefulness of the high-precision guide-block system for ultrahigh-pressure generation.

2.2. The first-stage anvils

The first-stage anvils are made of hardened steel in order to compress a KMAP assembly in combination with the second-stage WC anvils. As reported in Refs. [10], [11], the pressure increases rapidly under press loads of up to 4 MN, and then gradually under higher press loads at least up to 15 MN. Therefore, it is useful for a hydraulic system and press chassis to allow the application of press loads up to 15 MN. The second-stage anvils are frequently broken during ultrahigh-pressure generation, which limits their practical use in high-pressure research. Hence, the size of second-stage anvils should be minimized. Assuming that the compressional strength of hardened steel for second-stage anvils is 2 GPa, the truncated edge length (TEL) of first-stage anvils should be 50 mm. Thus, first-stage anvils usually have this TEL. In order to fit the TEL of first-stage anvils, second-stage anvils are usually around 26 mm in edge length.

2.3. The second-stage anvils

Needless to say, a harder anvil material allows higher pressure generation. Therefore, high-pressure generation in a KMAP significantly depends on the mechanical properties of the WC adopted for the second-stage anvils. Table 1 [20], [21] lists the mechanical properties of various grades of WC. Conventional WC anvils have a Vickers hardness (HV) of less than HV = 2000, which can only generate pressures up to 25 GPa [3]. In this work, we used WC with a high hardness of HV > 2000. Although these WC anvils can easily be broken by blow-out during decompression, their use is essential for generating ultrahigh pressures above 30 GPa. The TEL of the second-stage anvils is another important parameter in generating ultrahigh pressure, because the efficiency of pressure generation increases with decreasing TEL size. We empirically decided to use a TEL size of 1.5 mm. Higher pressures could be generated if smaller truncations were adopted.

Table 1. Mechanical properties of WCs.

Company	Grade	Vickers hardness, HV	Rockwell hardness, HRA	Compressive strength (GPa)
Tungaloy	F	1950a	93.4a	7.0a
Hawedia	HA-7% Co	1770a	93.0a	6.8a
Fujiloy	TF05	2400 [20]	95.1 [21]	> 8.0 [21]
Fujiloy	TJS01	2700 [20]	97.8 [21]	> 8.0 [21]

a: These are in the catalogues of the producing companies.

Pressure generation using any anvil material is limited by the anvil yielding, particularly around a truncation. If the compression space formed by second-stage anvils is not decreased due to yielding of the truncated corners of the anvils, the sample pressure does not increase despite the addition of a press load. We introduced tapering to the anvils in order to reduce this yielding. Three anvil faces around a truncation of second-stage anvils were tapered by 1°, which was determined empirically (Fig. 2(a)). This is essentially the same technology as bevel processing in DACs, by which pressures greater than 200 GPa were first achieved in a DAC [22]. Fig. 2(b) and Fig. 2(c), respectively, show schematic cross-sections of anvil tops for flat and tapered anvils under pressure. The anvil-tapering technique was pioneered in a KMAP about 40 years ago [23]. However, the pressure generation of that system was limited to about 30 GPa due to anvil hardness limitation at that time. Using IRIS-15, we conducted pressure generation tests at room temperature for flat and 1° tapered anvils with a truncation of 1.5 mm (Grade TF05) (Fig. 3). The pressures against the press load were determined based on changes in electric resistance corresponding to the following phase transitions: zinc sulfide (ZnS) semiconductor–metal (15.6 GPa [1], [24]), gallium phosphide (GaP) semiconductor–metal (23 GPa [1], [24]), zirconium (Zr) α–ω (8 GPa [25], [26]), and Zr ω–β (34 GPa [25], [26]). The press load for the ω–β transitions of Zr was reduced by 30% (from 10.8 to 7.5 MN) by the adoption of anvil tapering; higher efficiency in pressure generation is achieved by anvil tapering at relatively high press loads.

2.4. Cell assembly

Cell assembly setup is another important factor in the generation of ultrahigh pressures. Fig. 4 shows cross-sections of the cell assemblies used for high-pressure generation tests at room temperature and high temperature. An octahedron with edge lengths of 5.7 mm made of magnesium oxide (MgO) doped with 5 wt% of chromium(III) oxide (Cr2O3) was adopted for a pressure medium. A sample was placed at the center of the pressure medium. High pressures are generated by the volume decrease of a sample chamber. Therefore, if the materials in a high-pressure cell have high compressibility, the pressure increase is limited. For this reason, the sample was sandwiched with aluminum oxide (Al2O3) rods, because Al2O3 has a higher bulk modulus(∼240 GPa [27]) than MgO (∼160 GPa [27]).

For cell assembly for high-temperature experiments (Fig. 4(b)), a cylindrical rhenium (Re) or molybdenum (Mo) foil was adopted as a heater that could directly accommodate the sample material in the form of a sample capsule. The lighter element, Mo, was used as a heater for in situ X-ray diffraction experiments due to its higher X-ray transparency (Fig. 4(c)). Mo electrodes were used to electrically connect the heater and the second-stage anvils. At high temperatures, softening of the gaskets and pressure media causes pressure drops, because it prompts material flows through anvil gaps, and decreases the amount of materials confined in the compression spaces. We suppressed this softening by replacing significant parts of the Cr2O3-doped MgO pressure medium outside of the furnace with a LaCrO3 thermal insulator. Temperatures were measured at the surface of the heater with a W97%Re3%–W75%Re25% thermocouple that was electrically insulated from the LaCrO3 by Al2O3 tubes. For in situ X-ray diffraction experiments, Al2O3 X-ray windows with a diameter of 0.5 mm were set along the X-ray path outside of the furnace to suppress X-ray absorption by the pressure medium of Cr2O3-doped MgO and the thermal insulator of LaCrO3 (Fig. 4(c)). As shown later, however, these X-ray windows greatly decrease the sample pressure.

2.5. Pressure determination for in-house and in situ experiments and sample preparation

In conventional in-house experiments, generated pressures are estimated based on pressure calibration against the press load through the detection of phase transitions. However, one of the problems affecting KMAPs for pressures above 30 GPa is the irreproducibility of generated pressures against the press load, especially at high temperatures. For this reason, the pressures must be evaluated during each run. Since the Al2O3 content in bridgmanite (Brg, MgSiO3) coexisting with corundum (Cor, Al2O3) increases with pressure [28], generated pressures were estimated by measuring the composition of aluminous Brg in a run product after recovery using an electron microprobeanalyzer.

A sample with the composition Mg3Al2Si3O12, which is known as pyrope (Py), was used as the pressure calibrant. Py is phase stable at pressures above 2 GPa. At pressures higher than 26 GPa and at high temperatures, Py transforms to Brg (+ Cor), which results in a volume change greater than 10% [29] and causes a pressure drop. To suppress this pressure drop, we loaded sintered akimotoite with a Py composition (Py–Ak), which is one of the low-pressure phases of Brg and has a volume that is 8% smaller than that of Py. The synthesis of the Py–Ak was performed with an Mg3Al2Si3O12-compositional glass at 26 GPa and 1170 K [30]. In order to make the glass, an oxide mixture with the composition Mg3Al2Si3O12—prepared using MgO, Al2O3, and SiO2 oxides—was melted at 1950 K for 1 h, and then rapidly cooled in water. The synthesis of Py–Ak can be only conducted at relatively low temperatures up to 1170 K; this kind of phase has a high reactivity at higher pressures and temperatures. This pre-synthesis technique should be conducted for any samples in order to make a dense starting sample, which is synthesized at a lower temperature than the target temperature for an objective phase. In addition, the calibrant should be practically put together with a sample at a position where a thermocouple measures temperature.

A pressure calibrant for an in situ X-ray diffraction experiment was prepared by mixing sintered MgO with 5 wt% gold (Au) at 2 GPa and 1300 K for 1 h. Although these materials do not occur during any phase transition in our investigated pressure–temperature range, sintering is useful to suppress deformation of the sample part during compression and to efficiently generate high pressure due to low porosity.

3. Pressure generation at room temperature

Fig. 5 [10], [31] shows the results of pressure generation at ambient temperature by the technology described above using grade TF05 and TJS01 anvils. Generated pressures were determined by means of in situ X-ray diffraction with a pressure calibrant of Au, based on equations of state from Ref. [32]. Using TF05 anvils, we reached a pressure of 43 GPa at 15 MN, which was the maximal press load applicable. This pressure is much higher than that generated by conventional KMAP technology (∼25 GPa). Using TJS01 anvils, which are much harder than TF05 anvils, an even higher pressure of 64 GPa was achieved. This pressure is 2.5 times higher than that achieved using the conventional technique. If anvil tapering is not adopted, the pressure-increase rates will be close to zero under relatively high press loads (e.g., 0.2 GPa·MN−1above 9 MN) because of anvil deformation [11], [12]. However, the increase rate in our experiments remained at 1.5 GPa·MN−1 above 9 MN, thanks to the anvil tapering.

4. High-pressure generation at high temperature

High-pressure generation using TF05 anvils at 2000 K was examined based on the Al2O3 content in Brg (Fig. 6). At 6 MN, the aluminum (Al) number in Brg was 1.59(2) on the 12-oxygen basis, which suggests a generated pressure of 36 GPa. Furthermore, we synthesized a LiNbO3 (LN)-type phase with the Pycomposition [Al = 1.99(2)] [29] at 15 MN, which indicates a generated pressure of 45 GPa. Compared with pressure generation at ambient temperature, pressure generation at high temperature is more effective. We examined the effects of thermal insulation on pressure generation by in situ X-ray diffraction. When we used a cell assembly without a thermal insulator, a generated pressure of 43 GPa at ambient temperature dramatically decreased to 36 GPa at 1100 K; the experiment then failed by blow-out. This result is likely due to the softening of cell materials (e.g., the gasket and pressure medium), as mentioned in Section 2.3. Thus, efficient pressure generation at high temperature requires thermal insulation in order to maximize the heating efficiency and achieve a successful result.